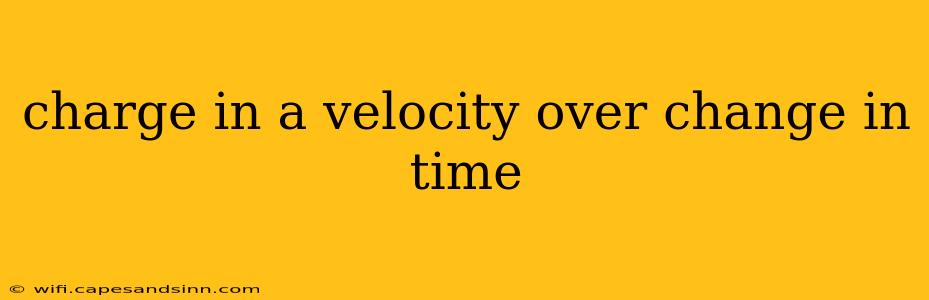
Understanding Acceleration: Charge in Velocity Over Change in Time
The phrase "charge in velocity over change in time" isn't standard physics terminology. However, it clearly alludes to the fundamental concept of acceleration. Acceleration is precisely the rate of change of velocity over time. Let's break down this crucial physics concept.
What is Acceleration?
In simple terms, acceleration describes how quickly an object's velocity is changing. Velocity itself encompasses both speed (how fast an object is moving) and direction. Therefore, an object accelerates if either its speed or its direction changes, or both.
Think of a car speeding up on a highway. Its speed is increasing, resulting in positive acceleration. If the car slows down to exit, it experiences negative acceleration, often called deceleration or retardation. Even a car driving at a constant speed around a curve is accelerating because its direction is constantly changing.
The Formula for Acceleration
The mathematical representation of acceleration is:
a = Δv / Δt
Where:
- a represents acceleration.
- Δv represents the change in velocity (final velocity - initial velocity).
- Δt represents the change in time (final time - initial time).
The units of acceleration are typically meters per second squared (m/s²) in the metric system or feet per second squared (ft/s²) in the imperial system.
Types of Acceleration
Understanding the different types of acceleration is crucial:
-
Uniform Acceleration: This occurs when the acceleration remains constant over time. A simple example is an object falling freely under gravity near the Earth's surface (neglecting air resistance).
-
Non-Uniform Acceleration: This is when the acceleration changes over time. A car accelerating from a standstill, gradually increasing its speed, is an example of non-uniform acceleration.
Beyond the Basics: Vectors and Acceleration
It's important to note that velocity and acceleration are vector quantities. This means they have both magnitude (size) and direction. Therefore, a change in direction alone constitutes acceleration, even if the speed remains constant.
This vector nature of acceleration is why circular motion, even at a constant speed, always involves acceleration towards the center of the circle (centripetal acceleration).
Real-World Applications of Acceleration
The concept of acceleration finds applications across various fields:
-
Engineering: Designing vehicles, rockets, and other moving machines requires a deep understanding of acceleration principles.
-
Physics: Analyzing the motion of projectiles, planets, and other celestial bodies relies heavily on acceleration calculations.
-
Sports Science: Analyzing athletic performance, optimizing training strategies, and understanding the biomechanics of movement often involve studying acceleration patterns.
Understanding acceleration is fundamental to grasping many areas of physics and engineering. While the phrase "charge in velocity" isn't formally used, the underlying concept – the rate of change of velocity over time – is central to the field. This detailed explanation aims to clarify the meaning and its significance in various applications.